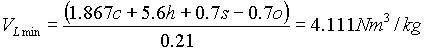
Example 6:  Ostwald Triangle and Bunte Diagram

A coal (c = 0,4028, h = 0,0322, n = 0,008, o= 0,1277, s = 0,029, w = 0,2323 i a = ?) burns in the furnace. 8%  of oxygen and 11%  of carbon dixide is measured in dry combustion products. Draw Ostwald triangle and Bunte diagram  for this coal and determine the excess of air land CO % . Apart of these, determine the excess of air approximately from O2% and from CO2 %.

Solution:

Minimal quantity of air needed for the combustion is:

A quantinty of dry combustion products if all carbon burns to CO2 is:
Maximal CO2 is:
A quantity of dry combustion products if all carbon burns to CO is:
VRS = VRSmin +0.9335c = 4.026+0.9335x0.402B = 4.402 Nm3/kg
Maximal CO is:
The unused oxigen is:
Maximal O2 in dry combustion products is 21%:
(O2)max=21%

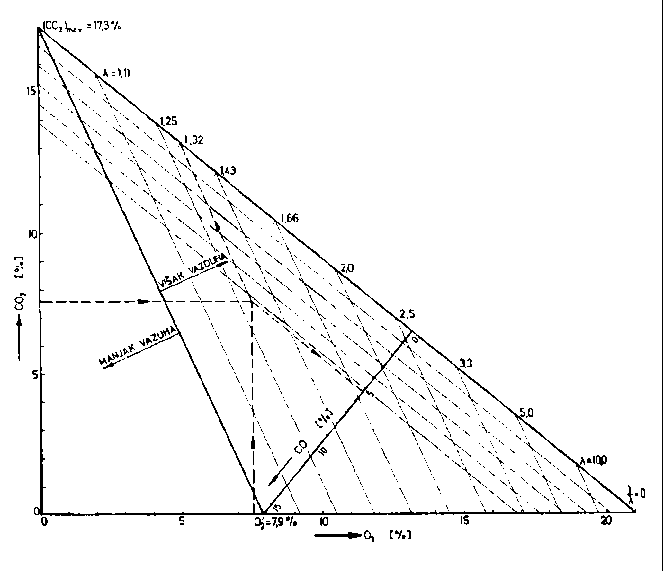
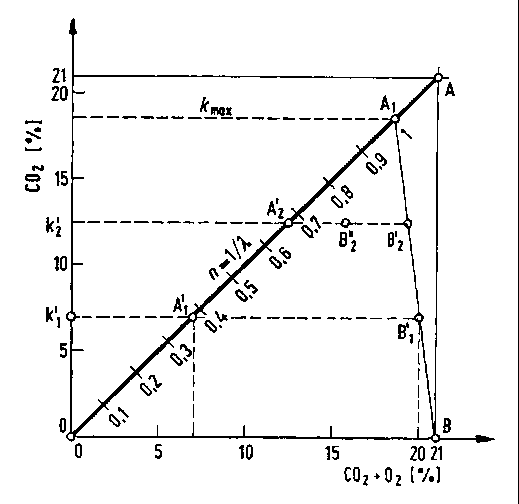
These percents are plotted on coordinate axes forming Ostwald triangle. The measured values are inserted into the diagram indicating that excess of air is 1.36 and CO is 5%.


Bunte diagram is plotted using a sum of  CO2 + O2 and CO2. A line with the slope of 45° is pointed to CO2max. Its length is devided proportionaly to 1/l.  For a measured CO2  AB" represents O2, while B"B'  is a content of CO. A scale of CO is obtained as follows:

Combustion of c to CO2

Combustion of c to CO gives:
V’RS = 1,867c + 0, 79 V’Lmin
which leads to:
Consequently, the scale of CO is 1,65 times the scale of CO2 i O2 .

The measured values of CO2 i O2 inserted into the diagram give l= 1,58, CO= 0,5%.

The excess of air determined by CO2 is:

and by O2: